Question

PLEASE HELP ME!! 30 points for right answer!

Solve the system of equations using linear combination and show your work.

g + h = 9
8g + 7.5h = 71

Answer 1

Given the system of equation:

g +h = 9 ......[1]

8g + 7.5h = 71 ......[2]

We can write equation [1] as;

g = 9-h ......[3]

Now, substitute equation [3] in [2] we get;

`8(9-h) + 7.5h = 71`

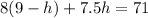
Using distributive property i,e
`a \cdot (b+c) = a\cdot b +a\cdot c`.

72 - 8h +7.5h = 71

Combine like terms:

72 - 0.5 h = 71

Subtract 72 to both sides of an equation:

72 - 0.5 h -72 = 71-72

Simplify:

- 0.5 h = -1

Divide both sides by -0.5 we get;

`(-0.5h)/(-0.5) = (-1)/(-0.5)`

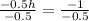
Simplify:

h = 2

Substitute the value of h =2 in equation [3] to solve for g;

g = 9 - 2 = 7

g = 7

Therefore, the solution for the given equation is :

g = 7 and h = 2