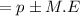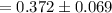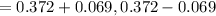Question

When 328 college students are randomly selected and surveyed, it is found that 122 own a car. find a 99% confidence interval for the true proportion of all college students who own a car?

Answer 1

`0.303<p<0.441`

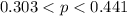
Explanation:

We know that,

`\text{Proportion}=p=(r)/(n)=(122)/(328)=0.372`

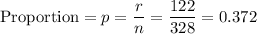
where

r = number of successful trials = 122,

n = 328

p = proportion

From probability distribution we also know that,

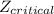`Z_(critical)` for a 99% confidence level = 2.576

Marginal error will be,

`M.E=Z_(critical)\cdot \sqrt{(p(1-p))/(n)`

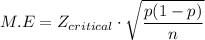
Putting the values,

`M.E=2.576\cdot \sqrt{(0.372(1-0.372))/(328)}=0.069`

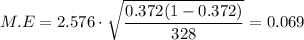
So the interval will be,

`=p\pm M.E`

`=0.372\pm 0.069`

`=0.372+ 0.069,0.372- 0.069`

`=0.441,0.303`