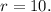Answer:
Answer in the form
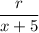 is
is
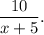
Explanation:
1 step: Multiply
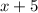 by
by
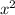 to get
to get
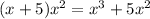
and subtract the result from the polynomial

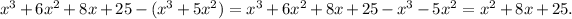
2 step: Multiply
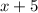 by
by
 to get
to get
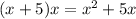
and subtract the result from the polynomial
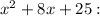

3 step: Multiply
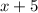 by
by
 to get
to get
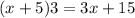
and subtract the result from the polynomial
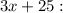

Thus, the remainder is