Question

HELP PLEASE.

Predict the new element formed when u-238 undergoes 2 alpha decay, 1 beta decay, and 1 gamma radiation. Write nuclear equation.

Answer 1

This hypothetical process would produce actinium-230.

Step-by-step explanation

An alpha decay reduces the atomic number of a nucleus by two and its mass number by four.

There are two types of beta decay: beta minus β⁻ and beta plus β⁺.

The mass number of a nucleus stays the same in either process. In β⁻ decay, the atomic number increases by one. An electron e⁻ is produced. In β⁺ decay, the atomic number decreases by one. A positron e⁺ is produced. Positrons are antiparticles of electrons.

β⁻ are more common than β⁺ in decays involving uranium. Assuming that the "beta decay" here refers to β⁻ decay.

Gamma decays do not influence the atomic or mass number of a nucleus.

Uranium has an atomic number of 92. 238 is the mass number of this particular isotope. The hypothetical product would have an atomic number of 92 - 2 ⨯ 2 + 1 = 89. Actinium has atomic number 89. As a result, the product is an isotope of actinium. The mass number of this hypothetical isotope would be 238 - 2 ⨯ 4 = 230. Therefore, actinium-230 is produced.

The overall nuclear reaction would involve five different particles. On the reactant side, there is

• one uranium-238 atom.

On the product side, there are

• one actinium-230 atom,
• two alpha particles (a.k.a. helium-4 nuclei),
• one electron, and
• one gamma particle (a.k.a. photon).

`\;_{\phantom{2}92}^(238) \text{U} \to \;_{\phantom{2}89}^(230) \text{Ac} + \;_(2)^(4) \text{He} + \;_(2)^(4) \text{He} + \text{e}^(-) + \gamma`

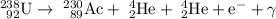
Consider: what would be the products if the nucleus undergoes a β⁺ decay instead?