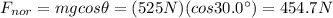Question

A 525-N trunk is placed on an inclined plane that forms an angle of 30.0° with the horizontal. What are the components of the weight parallel to the plane and normal to the plane?

Answer 1

262.5 N, 454.7 N

Step-by-step explanation:

The component of the weight parallel to the inclined plane is given by:

`F_(par)=mg sin \theta`

where

(mg) is the weight of the trunk

`\theta` is the angle of the ramp

In this problem,
`(mg)=525 N` while
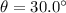`\theta=30.0^(\circ)`, so t he component of the weight parallel to the inclined plane is

`F_(par)=(525 N)( sin 30.0^(\circ))=262.5 N`

Instead, the component of the weight normal to the plane is given by:

`F_(nor)=mg cos \theta =(525 N)(cos 30.0^(\circ))=454.7 N`