Answer: 1,020 words
Explanation:
1. First, you have to use the same units to solve the problem. In the problem, both minutes and a half hour is given. They are different units for time. Choose one to solve the problem. It is easier to use the smaller units, which will be minutes. How many minutes are in an hour? 60 minutes are in an hour. A half hour will be half of the 60 minutes. Half of 60 minutes = 30 minutes, or: 60 ÷ 2 = 30.
2. Now that you know you need to use 30 minutes in the problem, you can set up your equation to solve.
(34 words÷minute) (30 minutes) = answer
3. There are two parts to solving a problem when units are involved. You have to solve for the numbers in the problem. You also have to solve for the units in the problem.
4. Let's take care of the units part first. People think it is hard, but it is not really. In this problem, we have:
words ÷ minutes × minutes
or
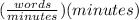
Since minutes are in the denominator of the fraction, or divided (either way you want to look at it), and it is also in the numerator of the fraction, or multiplied (either way you want to look at it), then they cancel each other out, or you can also say minutes÷minutes = 1, since any number (or in this case, units) divided by itself equals 1.
That leaves: words × 1 , which we know equals just words.
So: words × 1 = words
That takes care of your units part of the problem.
5. This leaves the numbers part of the problem, which everyone finds is easier to solve than the units. That's why we got the units out of the way first. We are now left with:
34 × 30 = ?
Which of course, the answer is 1020.
6. Now all of the problem put together:
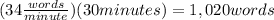
7. Therefore, the answer is: 1,020 words.