Question

Two runners start simultaneously from the same point on a circular 200-m track and run in opposite directions. One runs at a constant speed of 6.50 m/s , and the other runs at a constant speed of 5.00 m/s.

Required:
a. When will the fast one first overtake ("lap") the slower one?
b. How far from the starting point will each have run at that instant?
c. When will the fast one overtake the slower one for the second time?
d. How far from the starting point will they be at that instant?

Answer 1

17.4 s

Slower runner = 87 m, Faster runner = 113 m

34.78 s

Slower runner = 87 m, Faster runner = 113 m

Step-by-step explanation:

When the runners will cross each other they both would have run the same amount of time
`t`.

The total distance they both will cover when they will meet for the first time will be 200 m.

Velocity of the slow runner is 5 m/s and the other runner is 6.5 m/s.

Distance is given by
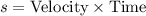`s=\text{Velocity}*\text{Time}` so

`5t+6.5t=200\\\Rightarrow t=(200)/(11.5)\\\Rightarrow t=17.4\ \text{s}`

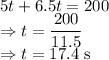
Both runners would have been running for 17.4 s when they would have met.

Distance covered by the slow runner is
`5* 17.4=87\ \text{m}`

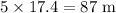
Distance covered by the other runner is
`200-87=113\ \text{m}`

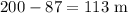
For the second time total distance they both will cover when they will meet for the first time will be 400 m.

`5t+6.5t=400\\\Rightarrow t=34.78\ \text{s}`

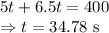
Both runners would have been running for 34.78 s when they would have met for the second time.

The runners will meet at the same distance from the starting point as they did the first time but the distances they cover would be double the distance they ran the first time.