Question

An elementary school class ran one mile with a mean of 12 minutes and a standard deviation of three minutes. Rachel, a student in the class, ran one mile in seven minutes. A junior high school class ran one mile with a mean of nine minutes and a standard deviation of two minutes. Kenji, a student in the class, ran 1 mile in 8.5 minutes. A high school class ran one mile with a mean of seven minutes and a standard deviation of four minutes. Nedda, a student in the class, ran one mile in eight minutes.

Required:
a. Why is Kenji considered a better runner than Nedda, even though Nedda ran faster than he?
b. Who is the fastest runner with respect to his or her class? Explain why.

Answer 1

a) Since Kenji's time has a lower Z-score's than Nedda, he is considered a better runner relative to his competition.

b) Since her time has the lowest Z-score, Rachel is the fastest runner with respect to her class.

Explanation:

Z-score:

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

a. Why is Kenji considered a better runner than Nedda, even though Nedda ran faster than he?

We have to see their z-scores.

Whoever has the lower z-score is in the lower percentile of times, that is, runs faster.

Kenji:

A junior high school class ran one mile with a mean of nine minutes and a standard deviation of two minutes. Kenji, a student in the class, ran 1 mile in 8.5 minutes.

So Kenji's z-score is found when
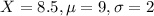`X = 8.5, \mu = 9, \sigma = 2`. So

`Z = (X - \mu)/(\sigma)`

`Z = (8.5 - 9)/(2)`

`Z = -0.25`

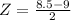
Nedda:

A high school class ran one mile with a mean of seven minutes and a standard deviation of four minutes. Nedda, a student in the class, ran one mile in eight minutes.

So Nedda's z-score is found when
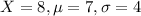`X = 8, \mu = 7, \sigma = 4`. So

`Z = (X - \mu)/(\sigma)`

`Z = (8 - 7)/(4)`

`Z = 0.25`

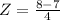
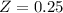
Since Kenji's time has a lower Z-score's than Nedda, he is considered a better runner relative to his competition.

b. Who is the fastest runner with respect to his or her class? Explain why.

Whoever has the lower z-score.

We have the z-scores for Kenji and Nedda, and we have to find Rachel's z-score.

An elementary school class ran one mile with a mean of 12 minutes and a standard deviation of three minutes. Rachel, a student in the class, ran one mile in seven minutes.

So Rachel's z-score is found when
`X = 7, \mu = 12, \sigma = 3`

`Z = (X - \mu)/(\sigma)`

`Z = (7 - 12)/(3)`

`Z = -1.67`

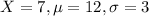
Since her time has the lowest Z-score, Rachel is the fastest runner with respect to her class.