Answer:
a. Q = 1881.73 x
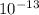 C
C
b. As battery is not removed so, potential difference will remain same.
c. E = 21.42 x
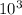 V/m
V/m
d. Q = 895.5 x
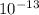 C
C
e. Again the potential difference will not change it will remain same as 9 V
f. E = 45 x
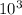 V/m
V/m
Step-by-step explanation:
Solution:
Here, Teflon is used so, the dielectric constant of the Teflon K = 2.1
Diameter = 2.1 cm
Radius = 2.1/2 cm
Radius = 1.05 cm
Radius = 0.015 m
Now, we need to find the area of each plate:
A =

A = (3.14) (
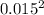 )
)
A = 0.000225

A = 2.25 x
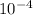

We are given the thickness of the plate which equal to the distance between the two plates.
d = 0.20 mm = 0.2 x
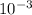 m
m
d = 0.2 x
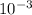 m = distance between two plates.
m = distance between two plates.
Hence, the capacitance of the dielectric without the dielectric
C =

Putting up the values we get,
E = 8.85 x
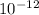
C =
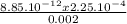
C = 99.5
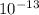
If dielectric is included then,
 = K C
= K C
 = (2.1) ( 99.5 x
= (2.1) ( 99.5 x
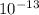 )
)
 = 209.08 x
= 209.08 x
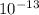 F
F
As we know the voltage of the battery V = 9V So,
a) Charge before the Teflon is removed:
Q = CV
Q =
 V
V
Q = (209.08 x
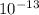 F) (9V)
F) (9V)
Q = 1881.73 x
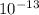 C
C
b) Potential Difference before the Teflon is removed = ?
As battery is not removed so, potential difference will remain same.
c) Electric Field =?
As we know,
E = V/(K.d)
E = 9V/(2.1 x 0.2 x
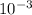 )
)
E = 21.42 x
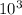 V/m
V/m
d) After the Teflon is removed
Q = CV
Q = (99.5
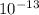 ) ( 9)
) ( 9)
Q = 895.5 x
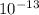 C
C
e) Again the potential difference will not change it will remain same as 9 V
f) Electric Field = ?
E =
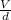 (Teflon is removed)
(Teflon is removed)
E = 9/0.2 x
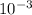
E = 45 x
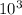 V/m
V/m