Question

Suppose of potassium bromide is dissolved in of a aqueous solution of silver nitrate. Calculate the final molarity of potassium cation in the solution. You can assume the volume of the solution doesn't change when the potassium bromide is dissolved in it. Round your answer to significant digits.

Answer 1

`M_(K^+)=0.0461M`

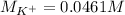
Step-by-step explanation:

Hello!

In this case, as the amounts are not given, we can assume the following problem that is similar to this one:

"Suppose 0.549g of potassium bromide is dissolved in 100.mL of a 19.0mM aqueous solution of silver nitrate. Calculate the final molarity of bromide anion in the solution. You can assume the volume of the solution doesn't change when the potassium bromide is dissolved in it. Round your answer to 3 significant digits."

In such a way, we consider that the molarity of any substance is computed by dividing the moles of solute and the volume of the solution; it means we need to compute the moles of potassium bromide in 0.549 g considering its molar mass is 119.002 g/mol:

`n_(KBr)=0.549gKBr*(1molKBr)/(119.002gKBr) =0.00461molKBr`

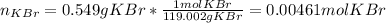
It also means that the moles of potassium cations are also 0.00461 mol as 1 KBr molecule has 1 potassium calcium. Moreover, given the volume of the silver nitrate solution, 100 mL, in L it would be 0.100 L, so the molarity of potassium ions turns out:

`M_(K^+)=(0.00461molKBr)/(0.100L)\\\\ M_(K^+)=0.0461M`

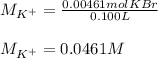
Best regards!