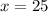Question

If the two acute angles of a right triangle have measures (2x-11) and (x+26) , then what is the value of X

Answer 1

ANSWER


`x = 25 \degree`

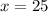
EXPLANATION


The sum of the interior angles of a triangle is

`180 \degree.`





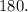
In a right angle triangle, one of the angles is already

`90 \degree.`



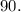
Therefore the remaining two acute angle will add up to

`90 \degree.`


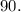
This implies that,


`(2x - 11) + (x + 26) = 90 \degree`



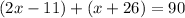
We group like terms to get,


`2x + x + 26 - 11 = 90`


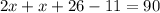
This gives us,


`3x + 15 = 90`


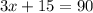
This gives,



`3x = 90 - 15`



`3x = 75`

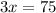
We divide both sides of the equation by 3 to obtain,


`x = 25 \degree`