Answer:
a) The probability that the thickness is less than 3.0 mm
P(X<3.0) = 0.057
b) The probability that the thickness is less than 7.0 mm
P(X>7) = 0.0401
c) The probability that the thickness is between 3.0 mm and 7.0 mm
P( 3 < x < 7)= 0.9029
Explanation:
Step(i):-
Given mean of the Population (μ) = 4.9 mm
Standard deviation of the Population(σ) = 1.2 mm
a)
Let 'X' be the random variable in normal distribution
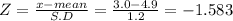
The probability that the thickness is less than 3.0 mm

= 1 - ( 0.5 + A(1.583)
= 0.5 - A(1.583)
= 0.5 - 0.4430
= 0.057
The probability that the thickness is less than 3.0 mm
P(X<3.0) = 0.057
b)
Let 'X' be the random variable in normal distribution
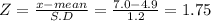
The probability that the thickness is less than 7.0 mm
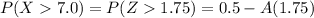
= 0.5 - 0.4599 ( from normal table )
= 0.0401
The probability that the thickness is less than 7.0 mm
P(X>7) = 0.0401
c)
Let 'X' be the random variable in normal distribution
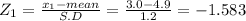
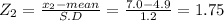
The probability that the thickness is between 3.0 mm and 7.0 mm
P( 3 < x < 7) = P( - 1.583 < X < 1.75 )
= A( 1.75 ) + A( -1.583)
= A(1.75)+A(1.583)
= 0.4599 + 0.4430
= 0.9029
The probability that the thickness is between 3.0 mm and 7.0 mm
P( 3 < x < 7)= 0.9029