Question

Rectangle ABCD is drawn on a grid with point A at (-3, 4), point B at (-1,4), point Cat (-1,-1) and point D at (-3,-1). If rectangle ABCD is reflected

over the line x = 2, what are the coordinates of the reflected rectangle A'B'C'D?

Options:

• A A75, 4). B'18. 4). C18.-1), D45.-1)

• B. A45.4), B (7,4), C(5.-1), D'(7.-1)

• C. A45, 4), B (7,4), C77, 1), D(5, 1)

• D. A(1,4). B(3,4), C13.-1). D'(1. -1)

Answer 1

Given:

The vertices of the rectangle ABCD are A(-3,4), B(-1,4), C(-1,-1) and D(-3,-1).

It is reflected over the line x=2.

To find:

The vertices of the image A'B'C'D'.

Solution:

If a figure is reflected over the line x=a, then

`(x,y)\to (-(x-a)+a,y)`

`(x,y)\to (-x+a+a,y)`

`(x,y)\to (-x+2a,y)`

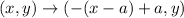
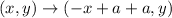
The rectangle ABCD is reflected over the line x=2. So, the rule of reflection is

`(x,y)\to (-x+2(2),y)`

`(x,y)\to (-x+4,y)`

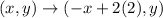
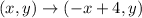
Using this rule, we get

`A(-3,4)\to A'(-(-3)+4,4)`

`A(-3,4)\to A'(3+4,4)`

`A(-3,4)\to A'(7,4)`

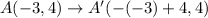
Similarly,

`B(-1,4)\to B'(5,4)`

`C(-1,-1)\to C'(5,-1)`

`D(-3,-1)\to D'(7,-1)`

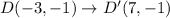
The vertices of image are A'(7,4), B'(5,4), C'(5,-1) and D'(7,-1).

Note: All options are incorrect or not in proper format.