Answer:
The rate of increase of x when A = 25 is 0.0006 cm/s.
Explanation:
Area of a square A =
 sq. cm
sq. cm
So, x =
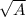 cm
cm
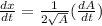 --- (1)
--- (1)
It is given that the area is increasing at the rate of 0.03
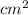 /s.
/s.
Therefore,
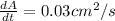
We need to find the rate of increase of x when A = 25.
Now, substituting the values of A and
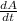 , (1) becomes
, (1) becomes
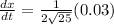
= 0.0006
Hence, the rate of increase of x when A = 25 is 0.0006 cm/s.