Question

A science teacher needs to choose 12 out of 16 students to serve as peer tutors how many different ways can the teacher choose the 12 students

Answer 1

8916100448256 is your answer like the other kid said

Answer 2

1820 ways.

Explanation:

We have been given that a science teacher needs to choose 12 out of 16 students to serve as peer tutors.

We will use combinations to solve our given problem.

The formula
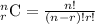`_(r)^(n)\textrm{C}=(n!)/((n-r)!r!)` represents number of ways to choose r items from n total items.

Upon substituting our given values in above formula, we will get:

`_(12)^(16)\textrm{C}=(16!)/((16-12)!12!)`

`_(12)^(16)\textrm{C}=(16!)/(4!*12!)`

`_(12)^(16)\textrm{C}=(16*15*14*13*12!)/(4*3*2*1*12!)`

`_(12)^(16)\textrm{C}=(4*5*7*13)/(1)`

`_(12)^(16)\textrm{C}=1820`

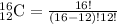
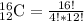
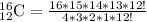
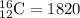
Therefore, the tutors can be chosen in 1820 different ways.