Question

Please answer this questions step by step​

Answer 1

(a) When a = 4 and b = -5, a² + b² - a·b = 61

(b) When a = 4 and b = -5, a³·b + a·b² = -220

(c)
`When \ a = 4 \ and \ b = -5, \ (a - b)/(a + b) + a -b = 0`

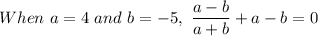
(d) When a = 4 and b = -5, (a + b)² - (a - b)² = 82

Explanation:

The question relates to evaluation of expressions, given the values of the variables, "a" and "b"

Where;

a = 4 and b = -5

(a) For a² + b² - a·b, we have;

a² + b² - a·b = 4² + (-5)² - 4 × (-5) = 16 + 25 + 20 = 41 + 20 = 61

(b) For a³·b + a·b² we have;

a³·b + a·b² = 4³·(-5) + 4·(-5)² = 64 × (-5) + 4 × 25 = -320 + 100 = -220

(c) For
`(a - b)/(a + b) + a -b`, we have;

`(a - b)/(a + b) + a -b = (4 - (-5))/(4 + (-5)) + 4 - (-5) = (4 + 5)/(4 -5) + 4 + 5= (9)/(-1) + 4 + 5 = -9 + 4 + 5 = 0`

`\therefore when \ a = 4 \ and \ b = -5, \ (a - b)/(a + b) + a -b = 0`

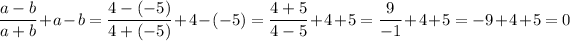
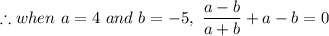
(d) For (a + b)² - (a - b)² we have;

(a + b)² - (a - b)² = (4 + (-5))² - (4 - (-5))² = (4 - 5)² - (4 + 5)² = (-1)² - 9² = 1 + 81 = 82

When a = 4 and b = -5, (a + b)² - (a - b)² = 82