Answer:
The slope is undefined because the x value is same and what we have will be a vertical line through the point x = -4
Explanation:
Here, we want to get the slope between the points (-4,-1) and (-4,3)
Mathematically, we can do this by using the slope equation below:
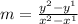
The coordinates with the subscript 1 represents the first point, while the coordinates with the subscript 2 represents the second point
We have that as follows:
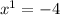
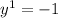
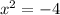
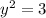
Now, we susbtitute the values into the slope equation above:
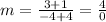
= Undefined.
The slope is undefined because the x value is same and what we have will be a vertical line through the point x = -4