Answer:
B. 3x + 5y = 1
Explanation:
Since, the equation of a line passing through
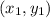 and
and
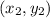 is,
is,

Thus, the equation of the line passes through the points (-3,2) and (2,-1) is,

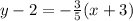
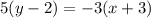
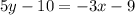
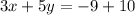
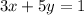
∵ Standard form of a line is ax + by = c, where, a, b and c are any constants,
Hence, the required equation is,
3x + 5y = 1
i.e. OPTION B is correct.