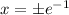Question

Solve for X


`lnx^(2) =-2`

please show steps

I have the answer,

I just want to know how to do this :)

Answer 1

Explanation:

lnx^n=nlnx

`\ln x^2=-2\\2\ln x=-2\\\\\ln x=-1\\\\x>0\\x=e^(-1)\\or~x=(1)/(e)`

Answer 2

`x = \pm e^(-1)`

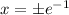
General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Equality Properties

• Multiplication Property of Equality
• Division Property of Equality
• Addition Property of Equality
• Subtract Property of Equality

Algebra I

• Solving quadratics
• Multiple roots

Algebra II

• Logarithms
• Euler's number e

Explanation:

Step 1: Define

`\displaystyle ln(x^2) = -2`

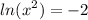
Step 2: Solve for x

1. Raise both sides by e:
   `e^(\displaystyle ln(x^2)) = e^(-2)`
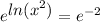
2. Simplify equation:
   `x^2 = e^(-2)`
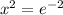
3. Square root both sides:
   `x = \pm e^(-1)`