The points are collinear, meaning that they are on the same line. Let's try to find the equation of the line. Since we have two points, we can use the point-slope form to find the equation, which is the following:
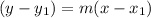
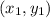 is a point on the line
is a point on the line
 is the slope of the line
is the slope of the line
First, let's find
 , the slope of the line. We are going to need to use the slope formula, which is the following:
, the slope of the line. We are going to need to use the slope formula, which is the following:
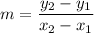
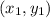 and
and
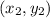 are points on the line
are points on the line
Applying this to our problem, we can find the slope:
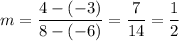
Now, let's find the equation of our line:
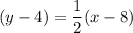
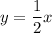
Now, let's set the equation of the line equal to 1, since we are trying to find the x-value that produces a y-value of 1.
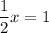
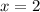
The answer is k = 2.