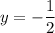Question

Find the horizontal asymptote of the graph of Y=(-3x^5+5x+4)/(6x^5+2x+5)

Answer 1

MrBillDoesMath!

Answer: y = -1/2

Discussion:

As x approaches plus or minus infinity the function behaves like

(-3x^5)/ (6x^5) = -1/2

MrB

Answer 2

Horizontal asymptote

`y=-(1)/(2)`

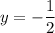
Explanation:

Given:
`y=(-3x^5+5x+4)/(6x^5+2x+5)`

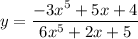
We need to find horizontal asymptote

For horizontal asymptote, we check the degree of numerator and denominator

Degree of numerator = 5

Degree of denominator = 5

Here degree is same

Therefore, Horizontal asymptote, y= leading coefficient of numerator over leading coefficient of denominator

Leading coefficient of numerator = -3

Leading coefficient of denominator = 6

Horizontal asymtote:

`y=(-3)/(6)=-(1)/(2)`

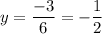
Hence, The horizontal asymptote is
`y=-(1)/(2)`