Question

I need help asap please

Answer 1

56π

Explanation:

1. Approach

To find the radius of a circle, use the formula;

`A = r^(2)`π

To find the area of the shaded region, find the area of the larger circle, and then subtract the area of the smaller circle from it.

It is given that the larger circle has a radius of 9, and the smaller has a radius of 5.

2. Area of the larger circle

`A = r^(2)`π

Substitute in known value (use 3.14 for π)

`A = (9)^(2) * 3.14`

A = 254.47

3. Area of the smaller circle

`A = r^(2)`π

Substitute in known value (use 3.14 for π)

`A = (5)^(2) * 3.14`

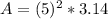
A = 78.54

4. Area of the shaded region

Subtract the area of the smaller circle from the area of the larger circle;

254.47 - 78.54 = 175.93

~ 56π