Question

Why is the product of two rational numbers always rational? Select from the drop-down menus to correctly complete the proof. Let ab and cd represent two rational numbers. This means a, b, c, and d are , and b and d are not 0. The product of the numbers is acbd , where bd is not 0. Both ac and bd are , and ​ bd ​ is not 0. Because ​ acbd ​ is the ratio of two , the product is a rational number.

Answer 1

is there any picture if not then i believe the answer is Integers, d is not zero, multiplication.

i don't know how to explain it though let me try to figure this out more if i find anything more out ill type it in the text!!

Answer 2

Answer: The proof is mentioned below.

Explanation:

Let a/b and c/d are two rational numbers where b ≠ 0 and d ≠ 0 ( by the property of rational number.) And, a, b, c and d are integers.

Proof that:
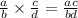`(a)/(b)* (c)/(d) = (ac)/(bd)` is also a rational number, for which bd≠ 0

Since a and b are integers therefore ab are also integers ( because integers are closed under multiplication)

Similarly cd is also an integer.

⇒
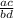`(ac)/(bd)` is a fraction in which both numerator and denominator are integers.

Moreover, b≠0 and d≠0 ⇒ bd≠0 ( because product of non zeros number is also non zero.)

Thus, by the property of rational number
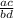`(ac)/(bd)` is also a rational number for which bd≠ 0

Therefore, The product of two rational is numbers always rational.