Question

Rewrite the equation y^2 - 2x + 2y-5=0 in standard form. Determine the focus and directrix

Answer 1

Answer : option D

`y^2 - 2x + 2y-5=0`

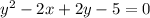
We apply completing the square method

Move all the y terms on one side

y^2 +2y = 2x + 5

In completing the square method , we take coefficient of y then divide it by 2 and square it

2/2 =1 then 1^2 = 1

Add it on both sides

`y^2 + 2y +1 = 2x+5+1`

`(y+1)^2= 2x+6`

`(y+1)^2= 2(x+3)`

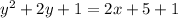
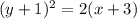
Solve for x

`(y+1)^2= 2x+6`

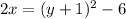`2x=(y+1)^2-6` (divide by 2 on both sides)

`x=1/2(y+1)^2-3`

Vertex is (h,k) that is (-3,-1). h= -3 and k = -1

The value of a= 1/2

`p = (1)/(4a)`

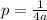
Plug in 1/2 for 'a'

so P = 1/2

Focus = (h+p, k)

h= -3 and k = -1, p = 1/2

`(-3+(1)/(2) , -1) = ((-5)/(2) , -1)`

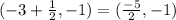
Directrix x=(h - P)

`x = -3 - (1)/(2) = (-7)/(2)`

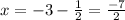
Option D is correct