Answer:

Explanation:
The equation of an ellipse outside the origin is as follows

The above is for an ellipse centered on (h, k). in this case the center is in (2,1) so
 and
and
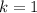
So far we have:
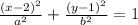
Now we find a and b, Where b is the semi-major axis and the minor semi-axis.
If the center is at (2, 1) and one focus is at (2,-2) this means there are 3 units of distance between the center and the focus. This quantity will be called c.
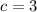
Now, of one vertex is at (2,-4) this means there are 5 units of distance between the center and the vertex, this is the semi major axis of the ellipse
 ⇒
⇒
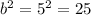
and to find a:
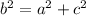
clearing for a:
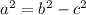
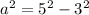

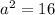
Substituting in the equation of the ellipse

wich is the second of the options.