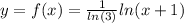Question

Determine the function which corresponds to the given graph.

a natural logarithmic function crossing the y axis at zero and going through the point 2,1.


The asymptote is x = -1.

Answer 1

The general natural logarithmic function
`y=ln(x)` has an asymptote at
`x=0`. To make this function have an asymptote at
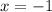`x=-1`, we need to translate the function 1 unit left. So the new function (according to rules of translations) takes the form

`y=ln(x+1)`

This function should also go through two points
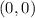`(0,0)` and
`(2,1)`. Let's check (0,0).

`ln(0+1)=ln(1)=0`. So the first point is okay. Let's check (2,1).

`ln(2+1)=ln3`, which is not equal to 1.

A simple trick to make this equal to 1, for us to satisfy all the conditions of making this function, is to think "What can we do to
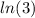`ln(3)` to make it equal to 1?"

Simple! We have to multiply it by
`(1)/(ln(3))`!

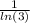Now, our final equation becomes
`y=(1)/(ln(3))ln(x+1)`.

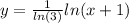ANSWER:
`y=f(x)=(1)/(ln(3))ln(x+1)`