Answer: (x + 1)(x - 2)(x + i√2)(x - i√2)
Explanation:
f(x) = x⁴ - x³ - 2x - 4
possible rational roots: +/- 1, 2, 4
Use Synthetic Division to find two of the roots (so you are left with a quadratic polynomial)
-1 | 1 -1 0 -2 -4
| ↓ -1 2 -2 4
1 -2 2 -4 0 → remainder is 0 so x = -1 is a root
⇒ (x + 1) is a factor
Now find another root using the decomposed polynomial:
2 | 1 -2 2 -4
| ↓ 2 0 4
1 0 2 0 →remainder is 0 so x = 2 is a root
⇒ (x - 2) is a factor
Now factor the decomposed polynomial:
x² + 0x + 2
= x² - (-2)
=
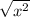 +/-
+/-
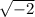
= (x + i√2)(x - i√2)