Question

Write the standard form of the equation of a circle with a radius of 2 and center at (4,-5)

Answer 1

`(x-4)^2+(y+5)^2=4`

Explanation:

We are given a circle with a radius of 2 with a center at the point (4, -5).

Assuming x and y to be the coordinates of any point on the circle, we can find the equation of the circle by using the following distance formula:

`r=√((x_1-x)^2+(y_1-y)^2)`

Putting the given values to get:

`2=√((x-4)^2+(y+5)^2)`

Taking square on both sides to get:

`4=(x-4)^2+(y+5)^2`

Therefore, the equation of the given circle with radius 2 and center at (4, -5) is
`(x-4)^2+(y+5)^2=4`.

Answer 2

We know that a circle is a locus of points which keep equal distance from a fixed point known as centre and the fixed distance known as radius.

Here given that centre is (4,-5) and radius =2

let (x,y) be any point on the circle.

Then by definition of circle, we have distance between (x,y) and cenre will be the constant = 2.

i.e. using distance formula

`√((x2-x1)^2+(y2-y1)^2) = √((x-4)^2+(y+5)^2) =2`

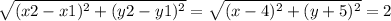
Square both the sides

[/tex]{(x-4)^2+(y+5)^2} =2^2[/tex]

Or

[/tex]{(x-4)^2+(y+5)^2} =4[/tex]

is the answer.