Question

The midsegment through the legs of an isosceles triangle is 4 in. Find the sides of the triangle if its perimeter is 20 in.

Answer 1

Given: Consider an isosceles triangle ABC as shown below in the attachment.

Perimeter = 20 in. and the mid segment through the legs of an isosceles triangle is 4 in.

An isosceles triangle is a triangle that has two sides of equal length.

Therefore, in triangle ABC, AB = AC = a (let) andf BC = b (let)

Construct a line DE parallel to BC.

The Triangle Mid segment Theorem states that in a triangle, the segment joining the midpoints of any two sides will be parallel to the third side and half its length.

As we know the mid segment is 4 in. i.e, DE = 4 in.

therefore, by mid segment theorem the parallel side i.e, BC = 2 DE

then; BC =
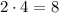`2 \cdot 4 = 8` in.

or BC = b = 8 in.

Perimeter of an isosceles triangle is the sum of the three lengths.

I,e P = a+a+b = 2a +b ; where P is the perimeter , a and b are the sides of the triangle.

Now, substituting the values of b = 8 in in the given perimeter formula to solve for a;

`2a+8 =20`

Subtract 8 from both sides of an equation;

2a+8-8 = 20 -8

Simplify:

2a = 12

Divide both sides by 2 we get;

`(2a)/(2) = (12)/(2)`

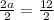
Simplify:

a =6 in.

Therefore, the sides of the given triangle is; a= 6 in and b = 8 in