For 14, I think the definition of the absolute value will make things easier. Recall that
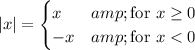
So we have
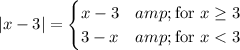
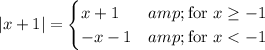
Putting these together, we have 3 different cases to consider:
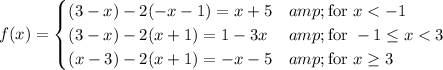
Then we check the derivative, noting that we shouldn't expect the derivative to be continuous at
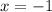 and
and
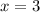 , so we ignore those exact cases:
, so we ignore those exact cases:
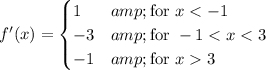
This tells us that
 is increasing on
is increasing on
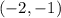 and decreasing on
and decreasing on
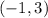 . We know that
. We know that
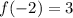 and
and
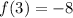 , but if we can verify that
, but if we can verify that
 is continuous at
is continuous at
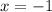 , then we can use the trends from the derivative test above to show there's at least a local maximum at that point.
, then we can use the trends from the derivative test above to show there's at least a local maximum at that point.
We have
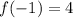 , while
, while
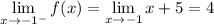

so
 is indeed continuous. So,
is indeed continuous. So,
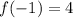 is an absolute maximum and
is an absolute maximum and
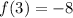 is an absolute minimum.
is an absolute minimum.
For 15, the fact that
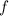 is differentiable and attains an extreme value at
is differentiable and attains an extreme value at
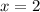 means that
means that
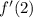 exists, and that
exists, and that
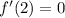 . Then for part (a),
. Then for part (a),
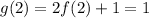

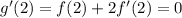
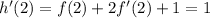
For part (b), in order for
 or
or
 to have extreme values at
to have extreme values at
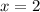 , we would need to have
, we would need to have
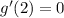 (which is true, as shown above) and
(which is true, as shown above) and
 (which is not).
(which is not).