Answer-

And cost of a car in the year 2000 will be $20134.18
Solution-
Let's assume,
x = number of years after 1986
y = average cost of sedan in dollar
The exponential model that will model the scenario will be in the form of,

where a and b are constants
As given that, in 1986 average cost of sedan was $9000 and in 1991 average cost of sedan was $12000
So, the points (0, 9000) and (5, 12000) will satisfy or lie on the exponential curve.
Putting (0, 9000) in the equation,
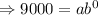

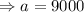
Now, the equation becomes
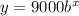
Putting (5, 12000) in this equation,
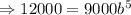
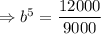
![\Rightarrow b=\sqrt[5]{(4)/(3)}](https://img.qammunity.org/2019/formulas/mathematics/high-school/hnryw2ig90slgt3dg7u9sf2nrrombih7aj.png)
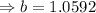
Putting the values,
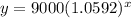
As we have to calculate the cost of sedan in 2000, so putting x=14(as 2000-1986=14),
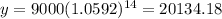
Therefore, cost of a car in the year 2000 will be $20134.18