Question

A basketball of mass 0.23kg is thrown horizontally against a rigid vertical wall with a velocity of 20m/s. It rebounds with a velocity of 15m/s. Calculate the impulse of the force of the wall on the basketball.

Answer 1

`8.1\:\mathrm{Ns}`

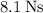
Step-by-step explanation:

The impulse-momentum theorem gives the impulse on an object to be equal to the change in momentum of that object. Since mass is maintained, the change in momentum of the basketball is:

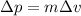`\Delta p = m\Delta v`, where
`m` is the mass of the basketball and
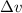`\Delta v` is the change in velocity.

Since the basketball is changing direction, its total change in velocity is:

`\Delta v = 20-(-15)=35\:\mathrm{m/s}`.

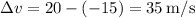Therefore, the basketball's change in momentum is:

`\Delta p = m\Delta v = 0.23\cdot 35= 8.05=8.1\:\mathrm{kg\cdot m/s}`.

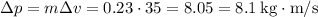Thus, the impulse on the basketball is
`\fbox{$8.1\:\mathrm{Ns}$}` (two significant figures).