Question

Jose asks his friends to guess the higher of two grades he received on his math tests. He gives them two hints. The difference of the two grades is 16. The sum of one-eighth of the higher grade and one-half of the lower grade is 52. The system that represents his scores is below. x – y = 16 1/8 x+ 1/2 y = 52 What is the higher grade of Jose’s two tests?

A. 48 B. 52 C. 80 D. 96

Answer 1

Option D. 96 is the correct answer.

Step-by-step explanation:

Let the higher grade be = x

Let the lower grade be = y

As given, The difference of the two grades is 16

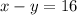`x-y=16` or
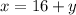`x=16+y` .... (1)

The sum of one-eighth of the higher grade and one-half of the lower grade is 52.

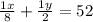`(1x)/(8)+(1y)/(2)=52` ... (2)

Putting the value of x=16+y in equation (2)

`(16+y)/(8)+(y)/(2)=52`

`(16+y+4y)/(8)=52`

`(16+5y)/(8)=52`

`16+5y=416`

`5y=400`

`y=80`

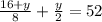
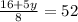
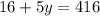
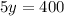
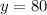
As x=16+y

x=16+80 = 96

Hence, higher grade is 96.