Question

Polygon ABCD is dilated, rotated, and translated to form polygon A′B′C′D′. The endpoints of AB are at (0, -7) and (8, 8), and the endpoints of A'B' are at (6, -6) and (2, 1.5). What is the scale factor of the dilation?

Answer 1

1/2

Explanation:

Answer 2

Given that there is a line segment ABwith end points at A(0.-7) and B(8,8)

this is transformed into A'B' with end points (6,-6) and (2,1.5)

To find the scla efactor of dilation.

It is enough to find the ratio of A'B' to AB.

AB = distance between A and B =
`√((8-0)^2+(8+7)^2) =√(64+225) =√(289) \\=17`

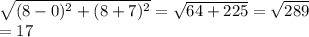
Let us find new length A'B'

A'B' = distance between A' and B' =
`√((2-6)^2+(1.5+6)^2) =√(16+56.25) =√(72.25) \\=8.5`

i.e. 17 distance is shrunk into 8.5 distance.

Hence scale factor= 8.5/17 = 1/2

there is a dilation of 1/2 .

Scale factor = 1/2