Question

How do I verify #15 using fundamental trig identities?

Answer 1

We start with the more complicated side which is the left side, and show that, on using some trigonometric identities, we will get the term on the right side .

`(sin \theta + tan \theta)/(1+cos \theta)`

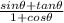
Using Quotient identity for tangent function, we will get

`(sin \theta+ (sin \theta)/(cos \theta))/(1+cos \theta)`

`(sin \theta cos \theta + sin \theta)/(cos \theta(1+cos \theta))`

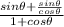
Taking out sine function from the numerator

`=(sin \theta(1+cos \theta))/(cos \theta(1+cos \theta))`

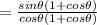
Cancelling the common term of numerator and denominator

`=(sin \theta)/(cos \theta) = tan \theta`