According to the Third Kepler’s Law of Planetary motion “The square of the orbital period of a planet is proportional to the cube of the semi-major axis (size) of its orbit”.
In other words, this law states a relation between the orbital period
 of a body (moon, planet, satellite) orbiting a greater body in space with the size
of a body (moon, planet, satellite) orbiting a greater body in space with the size
 of its orbit.
of its orbit.
This Law is originally expressed as follows:
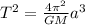 (1)
(1)
Where;
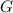 is the Gravitational Constant and its value is
is the Gravitational Constant and its value is
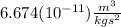
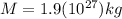 is the mass of Jupiter
is the mass of Jupiter
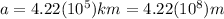 is the semimajor axis of the orbit Io describes around Jupiter (assuming it is a circular orbit, the semimajor axis is equal to the radius of the orbit)
is the semimajor axis of the orbit Io describes around Jupiter (assuming it is a circular orbit, the semimajor axis is equal to the radius of the orbit)
If we want to find the period, we have to express equation (1) as written below and substitute all the values:
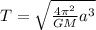 (2)
(2)
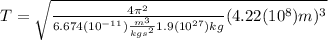

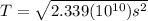
Then:
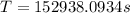 (3)
(3)
Which is the same as:

Therefore, the answer is:
The orbital period of Io is 42.482 h