Question

In a rally race the lead car is ahead by 1 1⁄2 hours. If the lead car is going 60 mph and the second place car is going 70 mph and starts 1 1⁄2 hours after the lead car, how long will it take to overtake the leader?

Answer 1

Let t hours be the time the second car is driving untill it overtakes the first car. The nfirst car is
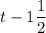`t-1(1)/(2)` hours on the road.

If the lead car is going 60 mph, then for the
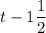`t-1(1)/(2)` hours it will go the distance

`60\cdot(t-1(1)/(2))\ m.`

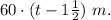
If the second place car is going 70 mph, then for the
hours it will go the distance

`70\cdot t\ m.`

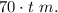
These distances are equal, then

`60(t-1(1)/(2))=70t,\\ \\60t-60\cdot (3)/(2)=70t,\\ \\10t=90,\\ \\t=9\ hours.`

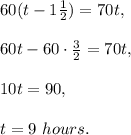
Answer: 9 hours