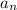Question

​ f(1)=72 f(n)=f(n−1)+9 ​ Find an explicit formula for f(n)

Answer 1

9n + 63

Explanation:

Answer 2

`a_(n)` = 9n + 63

generate the first few terms using the recursive equation

f(1) = 72

f(2) = 72 + 9 = 81

f(3) = 81 + 9 = 90

f(4) = 90 + 9 = 99

the sequence is 72, 81, 90, 99, .....

This is an arithmetic sequence whose n th term formula is

`a_(n)` =
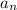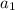`a_(1)` + (n - 1 )d

where
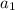`a_(1)` is the first term and d the common difference

d = 99 - 90 = 90 - 81 = 81 - 72 = 9 and
`a_(1)` = 72

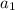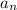`a_(n)` = 72 + 9(n - 1) = 72 + 9n - 9 = 9n + 63 ← explicit formula