Question

Find the m 33
43
57
67

Answer 1

What does the m stand for?

Answer 2

Answer: The correct option is

(A) 33°.

Step-by-step explanation: In the given figure, AB ║ CD and BC║ DE.

Also, m∠BCA = 90° and m∠BAC = 57°.

We are to find the measure of angle CDE.

We know that

the sum of the measures of the three angle of a triangle is 180°, so from triangle ABC, we have

`m\angle BCA+m\angle BAC+m\angle ABC=180^\circ\\\\\Rightarrow 90^\circ+57^\circ+m\angle ABC=180^\circ\\\\\Rightarrow m\angle ABC=180^\circ-90^\circ-57^\circ\\\\\Rightarrow m\angle ABC=180^\circ-143^\circ\\\\\Rightarrow m\angle ABC=33^\circ.`

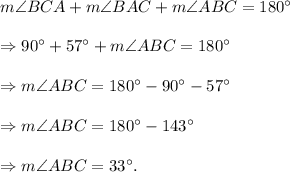
Now, since AB ║ CD and BC is a transversal so we get

`m\angle ABC=m\angle BCD~[\textup{alternate interior angles}]~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

Similarly, since BC║ DE and CD is the tranversal, so we get

`m\angle BCD=m\angle CDE~[\textup{alternate interior angles}]~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(ii)`

From equations (i) and (ii), we get

`m\angle CDE=m\angle ABC=33^\circ.`

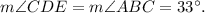
Thus, the required measure of angle CDE is 33°.

Option (A) is CORRECT.