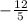Question

PLEASE HELP ME FIGURE THIS OUT 1....

Answer 1

Answer:
`(11)/(6)`

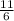
Explanation:

30°-60°-90° triangle has corresponding sides of: b - b√3 - 2b

sec²
`((\pi)/(3))` = sec² 60°

sec² 60° =
`(1)/(cos^(2)60)`

=
`((hypotenuse)/(adjacent))^(2)`

=
`((2b)/(b√(3)))^(2)`

=
`((2)/(√(3)))^(2)`

=
`(4)/(3)`

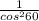
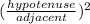
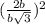
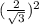
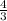
45°-45°-90° triangle has corresponding sides of: a - a - a√2

sin²
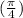`((\pi)/(4))` = sin² 45°

=
`((opposite)/(hypotenuse))^(2)`

=
`((a)/(a√(2)))^(2)`

=
`((1)/(√(2)))^(2)`

=
`(1)/(2)`

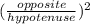
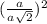
sec²
`((\pi)/(3))` + sin²
`((\pi)/(4))`

=
`(4)/(3)` +
`(1)/(2)`

=
`(4)/(3)((2)/(2))` +
`(1)/(2)((3)/(3))`

=
`(8+3)/(6)`

=
`(11)/(6)`

*********************************************************************************

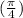
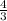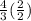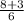
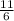
(-12, 5)

• the x-coordinate is the adjacent side = -12
• the y-coordinate is the opposite side = 5
• Use Pythagorean Theorem to find the hypotenuse = 13

(-12)² + (5)² = (hypotenuse)²

144 + 25 = (hypotenuse)²

169 = (hypotenuse)²

13 = hypotenuse

sin θ =
`(opposite)/(hypotenuse)` =
`(5)/(13)`

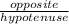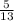
cos θ =
`(adjacent)/(hypotenuse)` =
`-(12)/(13)`

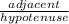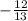
tan θ =
`(opposite)/(adjacent)` =
`-(5)/(12)`

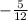
csc θ =
`(hypotenuse)/(opposite)` =
`(13)/(5)`

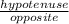sec θ =
`(hypotenuse)/(adjacent)` =
`-(13)/(12)`

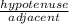cot θ =
`(adjacent)/(opposite)` =
`-(12)/(5)`