Question

Mike can build a go cart in 30 days, Jed can build one in 20 days and Erick in 60 days. If all of them share the work, how quickly can they build a go cart?

I know the answer is 10, but how do I get to that?

Answer 1

Together they build a cart in 10 days.

Explanation:

Given : Mike can build a go cart in 30 days, Jed can build one in 20 days and Erick in 60 days. If all of them share the work.

To find : How quickly can they build a go cart?

Solution :

Let the work done be in x days.

and total work done is 1.

Then, Mike can build a go cart in 30 days

i.e. Mike done his work in
`(x)/(30)`

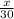
Jed can build one in 20 days

i.e. Jed done his work in
`(x)/(20)`

Erick can build one in 60 days

i.e. Erick done his work in
`(x)/(60)`

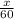
If they work together work done is 1.

i.e.
`(x)/(30)+(x)/(20)+(x)/(60)=1`

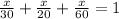
Taking LCM,

`(2x+3x+x)/(60)=1`

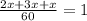
Cross multiply,

`6x=60`

`x=(60)/(6)`

`x=10`

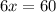
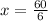
Therefore, Together they build a cart in 10 days.

Answer 2

Answer: 10 days

Explanation:

Mike:
`(x)/(30)`

Jed:
`(x)/(20)`

Erick:
`(x)/(60)`

Together:
`(x)/(30)` +
`(x)/(20)` +
`(x)/(60)` = 1

60
`((x)/(30)` +
`(x)/(20)` +
`(x)/(60)` = 1)

2x + 3x + x = 60

6x = 60

x = 10