1 Answer

Darronschall · Answer 1 · 2022-09-27T09:34:32+0000

Answer:

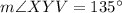
$m\angle XYV=135^(\circ)$

Explanation:

Notice that
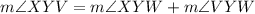$m\angle XYV=m\angle XYW+m\angle VYW$ .

The diagram already marks
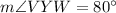$m\angle VYW=80^(\circ)$ , so we just need to find
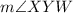$m\angle XYW$ .

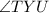$\angle TYU$ and
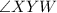$\angle XYW$ are vertical angles, which means they are equal in measure.

Therefore,

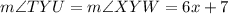
$m\angle TYU=m\angle XYW=6x+7$

Since there are
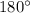$180^(\circ)$ on each side of a line, we have the following equation:

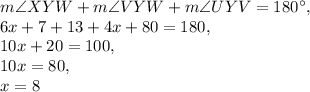$m\angle XYW+m\angle VYW+m\angle UYV=180^(\circ),\\6x+7+13+4x+80=180,\\10x+20=100,\\10x=80,\\x=8$ .

Plugging in
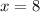x=8 , we have:

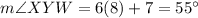$m\angle XYW=6(8)+7=55^(\circ)$ .

Therefore,

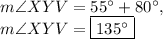$m\angle XYV=55^(\circ)+80^(\circ),\\m\angle XYV=\fbox{$135^(\circ)$}$ .

Please log in or register to add a comment.