Question

Which graph represents the piecewise-defined function?

{−4/5x−3   if  x<0
y= {
{3x−10   if  x≥2

Answer 1

it d for sure i just ddi the lesson

Step-by-step explanation:

there not right

Answer 2

Answer: The correct option is 1.

Step-by-step explanation:

The given piecewise function is,

`y=\begin{cases}-(4)/(5)x-3 & \text{ if } x<0\\3x-10 & \text{ if } x\geq2 \end{cases}`

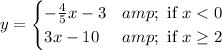
It means if x<0, then

`f(x)=-(4)/(5)x-3`

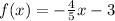
If
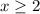`x\geq2`, then

`f(x)=3x-10`

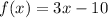
Since the f(x) is defined for x<0 and
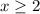`x\geq2`, therefore the function f(x) is not defined for
`0\leq x<2`.

From the graph 2, 3 and 4 we can easily noticed that for each value of x there exist a unique value of y, therefore the function is defined for all values of x, which is not true according to the given piecewise function.

Only in figure the value of y not exist when x lies between 0 to 2, including 0. It means the function is not defined for
`0\leq x<2`, hence the first option is correct.