Answer:
There are 9 ducks and 3 swans in the pond.
Explanation:
According to the problem, the number of swans in the pond is equal to the square root of the number of ducks, this can be expressed as
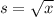
Where
 represents the number of ducks in the pond.
represents the number of ducks in the pond.
If the total number of swans and ducks is 12, the expression would be
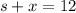
Now, we replace the first equation in the second one
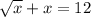
Then, we elevate each side to the square power
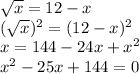
Using a calculator, the roots are 9 and 16.
Let's evaluate each value.
For
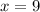 ,
,
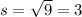 , and
, and
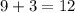 , which is true.
, which is true.
For
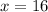 ,
,
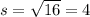 , and
, and
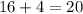 , which is false.
, which is false.
Therefore, there are 9 ducks and 3 swans in the pond.