Question

Assume that a procedure yields a geometric distribution where the probability of success is 4%. Find the probability that the first success occurs after the 19th trial.

Keyshawn is bowling in a competition. He has a 26% chance of getting a strike each time he bowls. What is the probability that Keyshawn gets a strike in his first 5 attempts?

Answer 1

For the first question I believe the answer is 46%

I'm honestly still looking for the second question's answer, so if you've already done the quiz or found the answer somewhere else would you mind giving it to me? I'd really appreciate it. If you haven't done it yet, I'll probably just put in the wrong answer and give it to you later

Answer 2

(A) Probability of success in a procedure=4 %

Probability of failure in the procedure = 100% - 4%=96%

Probability that the first success occurs after the 19th trial is equal to getting failure in first 19 attempts and then getting success.

As each trial is independent of another, so Probability is given by:

`=F^(19)* S\\\\=((96)/(100))^(19)* (4)/(100)\\\\= (0.96)^(19)* (0.04)\\\\ =0.46041 * 0.04\\\\=0.0184`

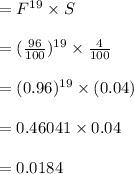
=1.84%

(B) Chances that Keyshawn will get a strike each time he bowls = 26%

And Probability that Keyshawn will not get a strike each time he bowls = 100%-26%=74%

Probability that Keyshawn gets a strike in his first 5 attempts=S F F FF + F S F FF + FF S FF+F F FSF+F F F F S

=5 × 4 failures × one Success

`=5* ((74)/(100))^4* (26)/(100)\\\\ =5 * 0.2998 * 0.26\\\\=0.3898`

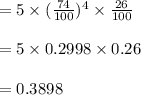
=0.39 or 39% (approximately)