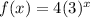Question

Which of these exponential functions goes through the points (1, 12) and (2, 36)?

A.) f(x) = 4(3)−x

B.) f(x) = 3(4)−x

C.) f(x) = 3(4)x

D.) f(x) = 4(3)x

Answer 1

Option D is correct

The exponential function is:
`f(x)= 4(3)^x`

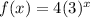
Step-by-step explanation:

An exponential function is in the general form is:

`f(x) =ab^x`. ......[1]

Given the points (1,12) and (2, 36).

For the point (1,12),

Plug in x = 1 and y =12 in [1]; we get

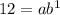`12=ab^1` or

12 =ab ......[2]

Now, for the point (2, 36);

Plug in x= 2 and y =36 in [1];

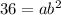`36=ab^2` ......[3]

Divide equation [3] by equation [2], we have

`(36)/(12)= (ab^2)/(ab)`

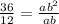
Simplify:

`3= b`

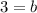
Substitute the value of b in equation [2], to solve for a;

`12 =a\cdot 3`

Divide both side by 3 we get;

`(12)/(3)= (3a)/(3)`

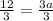
simplify:

`4 =a`

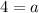
Then, put these a and b values in [1]; we get

The exponential function as:
`f(x)= 4(3)^x`