Question says that you have a cylindrical pan and a conical pan with the same height and radius.
Now we have to compare the volume of both type of pan and justify that using mathematical calculations.
Hypothesis:
I would say that cylindrical pan will have more volume than conical pan volume because of larger size.
Proof:
Given that diameter of both = 2 in
then radius r=diameter/2=2/2= 1 in
we can use formula of volume of each to find the volume of each can as shown below:
Volume of cylinder
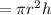
Volume of cylinder

Volume of cylinder

Volume of cone
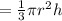
Volume of cone
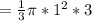
Volume of cone

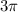 is clearly more than
is clearly more than
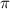
Hence volume of cylindrical pan is more than conical pan which proves that my hypothesis is correct.