Question

In △ABC, point M is the midpoint of AB , point D∈ AC so that AD:DC=2:5. If AABC=56 yd2, find ABMC, AAMD, and ACMD.

Answer 1

Answer: The area of triangle BMC is 28 yd square, the area of AMD is 8 yd square and the area of CMD is 20 yd square.

Step-by-step explanation:

Given information: M is the midpoint of the side AB. The line MC is the median of the triangle ABC.

A median divides the area of triangle in two equal parts, therefore the area of triangle BMC is half of the area of triangle ABC.

`\text{Area of }\triangle BMC=(1)/(2)* \text{Area of }\triangle ABC`

`\text{Area of }\triangle BMC=(1)/(2)* 56`

`\text{Area of }\triangle BMC=(1)/(2)* =28`

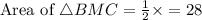
Therefore the area of BMC and AMC is 28 yd square.

Draw a perpendicular on AD from M as shown in the figure.

`\frac{\text{Area of }\triangle AMD}{\text{Area of }\triangle AMC}= ((1)/(2)* AD* ME)/((1)/(2)* AC* ME)`

`\frac{\text{Area of }\triangle AMD}{\text{Area of }\triangle AMC}=(AD)/(AC) =(2)/(7)`

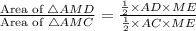
Therefore the area of AMD is
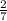`(2)/(7)`th part of the area of AMC.

`\text{Area of }\triangle AMD=(2)/(7)* \text{Area of }\triangle AMC`

`\text{Area of }\triangle AMD=(2)/(7)* 28=8`

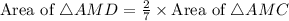
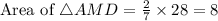
Therefore the area of AMD is 8 yd square.

`\text{Area of }\triangle CMD=\text{Area of }\triangle ABC-\text{Area of }\triangle AMD-\text{Area of }\triangle BMC`

`\text{Area of }\triangle CMD=56-8-28=20`

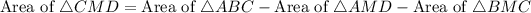
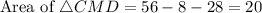
Therefore the area of CMD is 20 yd square.