Question

HURRY!!!! 20 POINTS!!!

Your local bakery sells more bagels when it reduces prices, but then its profit changes. The function y = -1000x^2 + 1100x -2.5 models the bakery’s daily profit in dollars from selling bagels, where x is the price of a bagel in dollars. What is the highest price the bakery can charge, in dollars, and make a profit of at least $200?

Answer 1

First step)

1. you must find the value of X for a profit of $ 200
2. then substitute the value of x i the equation

200= -1000x^2 + 1100x -2.5⇒ -1000x^2+1100x-2,5-200 = 0, ⇒-1000x^2 +110x-202,5 = 0

3. the point where the profit is highest is in he vertex of the parable for x = b/2a

x = - 1100/2(-1000) = 1100 /2000= 0,55, for this value the x, thah represent the price highest for a profit at least of $ 200

Answer 2

The highest price the bakery can charge, in dollars is $0.86623

Explanation:

We are given profit function as

`y=-1000x^2+110x-2.5`

where x is the price of a bagel in dollars

we are given least profit =200

so, we get inequality as

`-1000x^2+1100x-2.5\geq 200`

now, we have to find highest value of price or x

So, we will solve for inequality and then we choose largest value of x

Firstly, we set it equal and then we solve for x

`-1000x^2+1100x-2.5=200`

We will multiply both sides by 10

`-1000x^2\cdot \:10+1100x\cdot \:10-2.5\cdot \:10=200\cdot \:10`

`-10000x^2+11000x-25=2000`

`-10000x^2+11000x-2025=0`

we can use quadratic formula

`x=(-b\pm √(b^2-4ac))/(2a)`

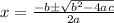
now, we can compare and find a,b and c

a=-10000 , b=11000, c=-2025

we get

`x=(-11000+√(11000^2-4\left(-10000\right)\left(-2025\right)))/(2\left(-10000\right))`

`x=(-11000-√(11000^2-4\left(-10000\right)\left(-2025\right)))/(2\left(-10000\right))`

`x=(11-2√(10))/(20),\:x=(11+2√(10))/(20)`

`x=0.2337,x=0.86623`

Firstly, we will draw a number line and locate these values

and then we can check sign of inequality on each intervals

so, we got interval as

`x:[0.2337,0.86623]`

now, we can find largest x-value

x=0.86623

So, the highest price the bakery can charge, in dollars is $0.86623