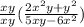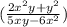Answer:
The simplified version of the given expression is
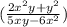
Explanation:
We are given an expression which is a complex fraction:

We can take LCM of these fractions to get:
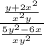
Taking the reciprocal of the lower fraction to change it to multiplication:
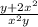 ×
×
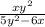
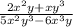
Taking xy as common from both the numerator and the denominator to get: